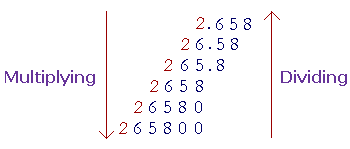|
Lesson 3 MULTIPLY BY POWERS OF 10DIVIDE BY POWERS OF 10The Meaning of PercentIn this Lesson we will learn to multiply and divide by a power of 10 -- simply by moving the decimal point. Having done that, we can then understand scientific notation. These are problems that do not require a calculator, and certainly should not be calculated in writing. In this Lesson, we will answer the following:
|
||||||||||||||||||||||
|
||||||||||||||||||||||
|
||||||||||||||||||||||
|
||||||||||||||||||||||
|
Examples.
Problem. If 5 pounds of sugar cost $2.79, how much will 50 pounds cost? Answer. Since 50 pounds are ten times 5 pounds, they will cost ten times more. Move the decimal point one place right: $27.90. Since money has two decimal places, we add on a 0. (Lesson 3, Question 8) |
||||||||||||||||||||||
|
||||||||||||||||||||||
Finally, we must see how to divide a whole number by a power of 10. In Lesson 1 we saw that when a whole number ends in 0's, we simply take off 0's. (Lesson 1, Question 11) 265,000 ÷ 100 = 2,650 But when a whole number does not end in 0's -- as in 265 -- then there are no 0's to chop off! We will see that we must separate digits from the right. First: |
||||||||||||||||||||||
|
||||||||||||||||||||||
|
||||||||||||||||||||||
|
||||||||||||||||||||||
Again, as in Lesson 1, consider this array:
As we move down the list -- as we push the digits one place left -- the number has been multiplied by 10, because the next place is worth 10 times more. (As we move from 2.658 to 26.58, we go from 2 ones to 2 tens.) It appears, though, as if the decimal point has shifted one place right, or, with whole numbers, that a 0 has been added on. As we move up the list -- as we push the digits to the right -- each number has been divided by 10. And so we can easily multiply or divide by a power of 10 because of the written system itself. Each place belongs to the next power of 10. |
||||||||||||||||||||||
|
||||||||||||||||||||||
|
2.345 That number is written in scientific notation. There is one digit to the left of the decimal point -- 2 -- and it is not 0. In general, a number written in scientific notation will be multiplied by 10 raised to an "exponent." 2.345 × 103 2.345 × 10−3 Without going into the details of what the exponents 3 and −3 actually mean, we can state the following : A positive exponent means to multiply by a power of 10. A negative exponent means to divide. Briefly, the exponent indicates the number of 0's Therefore, if a number is written in scientific notation, then to express it as a standard number, we can state the following rule: If the exponent is positive, move the decimal point right as many places as indicated by the exponent. If the exponent is negative, move the decimal point left as many places as indicated by the exponent. Example 1. Each number is written in scientific notation. What number is it? a) 5.42 × 103 = 5,420. Move the decimal point three places right. b) 5.42 × 10−3 = .00542 Move the decimal point three places left. Example 2. Write each number in scientific notation.
At this point, please "turn" the page and do some Problems. or Continue on to the next Section. Introduction | Home | Table of Contents www.proyectosalonhogar.com |
||||||||||||||||||||||