11

COMPLETING THE SQUARE

IF WE TRY TO SOLVE this quadratic equation by factoring

x² + 6x + 2  =  0

we cannot.  Therefore, we use a technique called completing the square.  This means to make the quadratic into a perfect square trinomial, i.e. the form  a² + 2ab + b²  =  (a + b)².

The technique is valid only when 1 is the coefficient of x².

1)  Transpose the constant term to the right:

x² + 6x = −2

2)  Add a square number to both sides.  Add the square of half the coefficient of x.  In this case, add the square of 3:

x² + 6x + 9 =  −2 + 9

The left-hand side is now the perfect square of  (x + 3).

(x + 3)²  =  7

3 is half of the coefficient 6.

This equation has the form

a²		=	b
which implies
a		=	±.
Therefore,
x + 3		=	±
x		=	−3 ±.

That is, the solutions to

x² + 6x + 2  =  0

are the conjugate pair,

−3 + ,  −3 − .

We can check this.  The sum of those roots is −6, which is the negative of the coefficient of x.  And the product of the roots is

(−3)² − ()²  =  9 − 7  =  2,

which is the constant term.  Thus both conditions on the roots are satisfied.  These are the two roots of the quadratic.

Problem.   Solve this quadratic equation by completing the square.

x² − 2x − 2 = 0

To see the solution, pass your mouse over the colored area.
To cover the solution again, click "Refresh" ("Reload").

x² − 2x	=	2
x² − 2x + 1	=	2 + 1
(x − 1)²	=	3
x − 1	=	±
x	=	1 ±

Before considering the quadratic formula, note that half of any

number b is

b 2

.   Half of  5 is

5 2

.   Half of

p q

is

p 2q

.

Theorem.   If

ax² + bx + c = 0,

Theorem.   then

To prove this, we will complete the square.  But to do that, the coefficient of x² must be 1.  Therefore, we will divide both sides of the original equation by a:

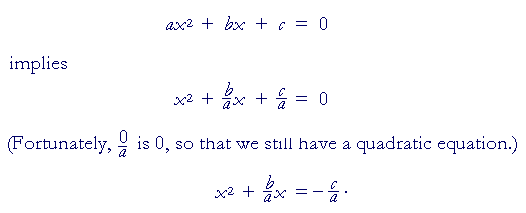
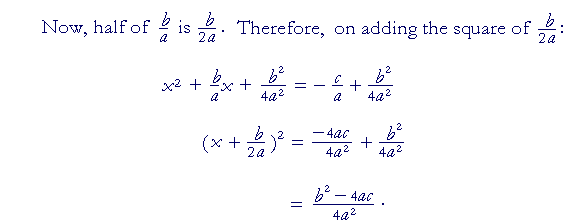
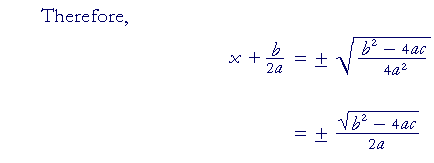
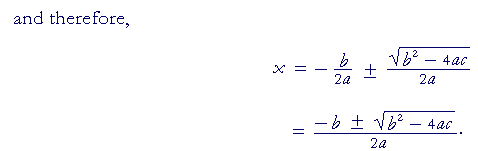
This is the quadratic formula.

Next Topic:  Synthetic division by x − a

Table of Contents | Home