16
SYMMETRY
Test for symmetry: Even and odd functions
LET THIS BE THE right-hand side of the graph of a function:
We will now draw the left-hand side -- so that the graph will be symmetrical with respect to the y-axis:
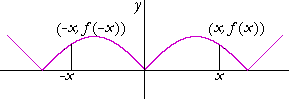
In this case,
f(−x) = f(x).
The height of the curve at −x is equal to the height of the curve at x -- for every x in the domain of f.
Again, let this be the right-hand side:

We will now draw the left-hand side -- so that the graph will be symmetrical with respect to the origin:

Every point on the right-hand side is reflected through the origin. In this case,
f(−x) = −f(x).
The height of the curve at −x is the negative of the height at x.
(A reflection through the origin is equivalent to a reflection about the y-axis, followed by a reflection about the x-axis.)
Test for symmetry of a function f(x)
Symmetry, then, depends on the behavior of f(x) on the other side of the y-axis -- at minus-x : f(−x).
To see the answer, pass your mouse over the colored area.
To cover the answer again, click "Refresh" ("Reload").
If f(−x) = f(x),
then f(x) is symmetrical with respect to the y-axis.
If f(−x) = −f(x),
then f(x) is symmetrical with respect to the origin.
Example 1. Test this function for symmetry:
f(x) = x4 + x² + 3
Solution. We must look at f(−x):
| f(−x) | = | (−x)4 + (−x)² + 3 |
| = | x4 + x² + 3 | |
| = | f(x) | |
Since f(−x) = f(x), this function is symmetrical with respect to the y-axis.
A function symmetrical with respect to the y-axis is called an even function.
Example 2. Test this function for symmetry:
f(x) = x5 + x³ + x
Solution. Again, we must look at f(−x):
| f(−x) | = | (−x)5 + (−x)³ + (−x) |
| = | −x5 − x³ − x | |
| = | −(x5 + x³ + x) | |
| = | −f(x) | |
Since f(−x) = −f(x), this function is symmetrical with respect to the origin.
A function that is symmetrical with respect to the origin is called an odd function.
Problem. Test each of the following for symmetry. Is f(x) even, odd, or neither?
a) f(x) = x³ + x² + x + 1
Answer. Neither, because f(−x) ≠ f(x) , and f(−x) ≠ −f(x).
b) f(x) = 2x³ − 4x
Answer. f(x) is odd -- it is symmetrical with respect to the origin -- because f(−x) = −f(x).
c) f(x) = 7x² − 11
Answer. f(x) is even -- it is symmetrical with respect to the y-axis -- because f(−x) = f(x).
Note: A polynomial will be an even function when all the exponents are even.
A polynomial will be an odd function when all the exponents are odd.
But there are even and odd functions that are not polynomials. Therefore, the issue is the test of f(−x).
www.proyectosalonhogar.com
