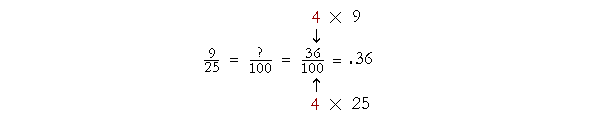|
Example 5. Write |
9
25 |
as a decimal. |
Solution. 25 is not a power of 10, but we can easily make it a power of 10 -- 100 -- by multiplying it by 4. We must also, then, multiply the numerator by 4.
|
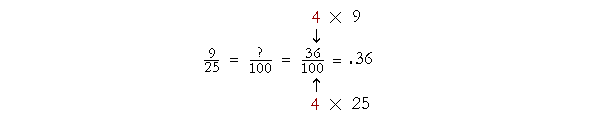
Example 6. Write |
4
5 |
as a decimal. |
We can make 5 into 10 by multiplying it -- and 4 -- by 2.
|
Example 7. Write as a decimal: |
7
200 |
| Answer. |
7
200 |
= |
35
1000 |
= .035 |
We can make 200 into 1000 by multiplying it -- and 7 -- by 5.
Alternatively, according to the properties of division,
7
200 |
= |
_ 7 _
2 × 100 |
= |
3.5
100 |
, on dividing 7 by 2, |
| |
| |
= |
.035, |
on dividing 3.5 by 100. |
|
Example 8. Write as a decimal: |
8
200 |
| Answer. |
8
200 |
= |
4
100 |
= .04 |
Here, we can change 200 into a power of 10 by dividing it by 2. We can do this because 8 also is divisible by 2.
Or, again,
8
200 |
= |
_ 8 _
2 × 100 |
= |
4
100 |
= .04 |
|
Example 9. Write as a decimal: |
12
400 |
| Answer. |
12
400 |
= |
3
100 |
= .03 |
We can change 400 to 100 by dividing it -- and 12 -- by 4.
To summarize: We go from a larger number to a smaller by dividing (Examples 8 and 9); from a smaller number to a larger by multiplying (Example 5).
Frequent decimals
The following fractions come up frequently. The student should know their decimal equivalents.
1
2 |
|
1
4 |
|
3
4 |
|
1
8 |
|
3
8 |
|
5
8 |
|
7
8 |
|
1
3 |
|
2
3 |
| Next, |
1
4 |
. But |
1
4 |
is half of |
1
2 |
. |
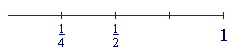
Therefore, its decimal will be half of .50 --
| And since |
3
4 |
= 3 × |
1
4 |
, then |
| Next, |
1
8 |
. But |
1
8 |
is half of |
1
4 |
. |
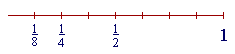
Therefore, its decimal will be half of .25 or .250 --
The decimals for the rest of the eighths will be multiples of .125.
Since 3 × 125 = 375,
| Similarly, |
5
8 |
will be 5 × |
1
8 |
= 5 × .125. |
5 × 125 = 5 × 100 + 5 × 25 = 500 + 125 = 625.
(Lesson 8) Therefore,
7 × 125 = 7 × 100 + 7 × 25 = 700 + 175 = 875.
Therefore,
These decimals come up frequently. The student should know how to generate them quickly.
The student should also know the decimals for the fifths:
The rest will be the multiples of .2 --
2
5 |
= |
2 × |
1
5 |
= 2 × .2 = .4 |
| |
3
5 |
= 3 × .2 = .6 |
| |
4
5 |
= 4 × .2 = .8 |
|
Example 10. Write as a decimal: 8 |
3
4 |
The whole number does not change. Simply replace the common
| fraction |
3
4 |
with the decimal .75. |
|
Example 11. Write as a decimal: |
7
2 |
Answer. First change an improper fraction to a mixed number:
"2 goes into 7 three (3) times (6) with 1 left over."
Example 12. How many times is .25 contained in 3?
| Answer. .25 = |
1
4 |
. And |
1
4 |
is contained in 1 four times. (Lesson 19.)
|
| Therefore, |
1
4 |
, or .25, will be contained in 3 three times as many times. It will |
be contained 3 × 4 = 12 times.
Example 13. How many times is .125 contained in 5?
| Answer. .125 = |
1
8 |
. And |
1
8 |
is contained in 1 eight times. Therefore, |
1
8 |
, |
or .125, will be contained in 5 five times as many times. It will be contained 5 × 8 = 40 times.
| As for |
1
3 |
and |
2
3 |
, they cannot be expressed exactly as a decimal. |
However,
1
3 |
 |
.333 |
And
2
3 |
|
.667 |
See Section 2, Question 3.
Frequent percents
From the decimal equivalent of a fraction, we can easily derive the percent: Move the decimal point two places right (Lesson 3.) Again, the student should know these. They come up frequently.
1
2 |
= |
.50 |
= |
50% |
| |
1
4 |
= |
.25 |
= |
25% |
| |
3
4 |
= |
.75 |
= |
75% |
| |
1
8 |
= |
.125 |
= |
12.5% (Half of |
1
4 |
.) |
| |
3
8 |
= |
.375 |
= |
37.5% |
| |
5
8 |
= |
.625 |
= |
62.5% |
| |
7
8 |
= |
.875 |
= |
87.5% |
| |
1
5 |
= |
.2 |
= |
20% |
| |
2
5 |
= |
.4 |
= |
40% |
| |
3
5 |
= |
.6 |
= |
60% |
| |
4
5 |
= |
.8 |
= |
80% |
In addition, the student should know
1
3 |
= 33 |
1
3 |
% |
| |
2
3 |
= 66 |
2
3 |
% |
(Lesson 15)
At this point, please "turn" the page and do some Problems.
or
Continue on to the next Section.
Introduction | Home | Table of Contents
www.proyectosalonhogar.com |
