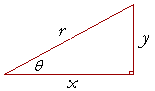
Proof of the reciprocal identities Proof of the tangent and cotangent identities Proof of the Pythagorean identities
The proof of each of those follows from the definitions of the trigonometric functions, Topic 16. Proof of the reciprocal relations By definition:
Therefore,
and vice-versa. Similarly for the remaining functions. Proof of the tangent and cotangent identities To prove:
Proof. By definition,
Therefore, on dividing both numerator and denominator by r,
These are the two identities. Proof of the Pythagorean identities To prove:
Proof. According to the Pythagorean theorem, x² + y² = r². . . . . . . . . . . . . . . .(1) Therefore, on dividing both sides by r²,
That is, according to the definitions, cos²θ + sin²θ = 1. Apart from the order of the terms, this is the first Pythagorean identity, a). To derive b), divide line (1) by x²; and to derive c), divide by y². Please make a donation to keep TheMathPage online. Copyright © 2001-2007 Lawrence Spector Questions or comments? E-mail: themathpage@nyc.rr.com |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
