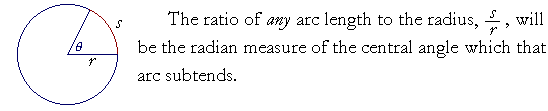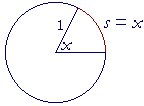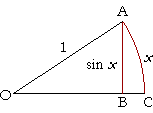15 Arc Length The definition of radian measure
IT IS CONVENTIONAL to let the letter s (for space) symbolize the length of an arc, which is called arc length. We say in geometry that an arc "subtends" an angle θ; literally, "stretches under." Now the circumference of a circle is an arc length. And the ratio of the circumference to the diameter is the basis of radian measure. That ratio is the definition of π.
Since D = 2r, then
or,
Thus the radian measure is based on ratios -- numbers -- that are actually found in the circle. The radian measure is a real number that indicates the ratio of a curved line to a straight, of an arc to the radius. For, the ratio of s to r does determine a unique central angle θ. In any circles the same ratio of arc length to radius 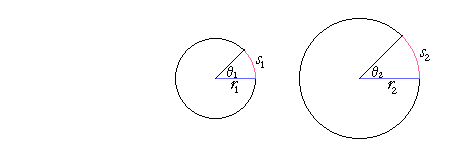
If, proportionally,
then θ1 = θ2. We will prove this theorem below.
radian measure of the central angle. At that central angle, the arc is four fifths of the radius. Example 2. An angle of .75 radians means that the arc is three fourths of the radius. s = .75r Example 3. In a circle whose radius is 10 cm, a central angle θ intercepts an arc of 8 cm. 
a) What is the radian measure of that angle? Answer. According to the definition:
b) At that same central angle θ, what is the arc length if the radius is 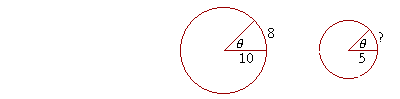
Answer. For a given central angle, the ratio of arc to radius is the same. 5 is half of 10. Therefore the arc length will be half of 8: 4cm. Example 4. a) At a central angle of 2.35 radians, what ratio has the arc to the radius? Answer. That number is the ratio. The arc is 2.35 times the radius. b) In which quadrant of the circle does 2.35 radians fall? 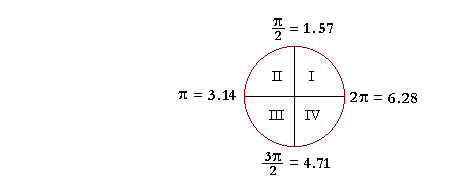
= 4.71. An angle of 2.35 radians, then, is greater than 1.57 but less that 3.14. It falls in the second quadrant. s = rθ If the radius is 10 cm, and the central angle is 2.35 radians, then how long is the arc? Answer. We let the definition of θ,
become a formula for finding s :
Therefore, s = 10 × 2.35 = 23.5 cm Because of the simplicity of that formula, radian measure is used exclusively in theoretical mathematics.
Once again: The radian measure is a real number x. And in the unit circle -- r = 1 -- the length of the arc s is that real number. s = rθ = 1· x = x. It is here that the term trigonometric "function" has its full meaning. For, corresponding to each real number x -- each radian measure, each arc -- there is a unique value of sin x, of cos x, and so on. The definition of a function is satisfied. (Topic 3 of Precalculus.) Thus, radian measure can be identified as the length x of an arc of the unit circle. Therefore when we draw the graph of y = sin x (Topic 19), we can imagine the unit circle rolled out in both directions onto the x-axis, thus labeling the x-axis. Because radian measure can be identified as an arc, the inverse trigonometric functions have their names. "arcsin" is the arc -- the radian measure -- whose sine is a certain number.
In the unit circle, the vertical side AB is sin x.
very small values of x. And we can see that when the point A is very close to C -- that is, when the central angle AOC is very, very small -- then the opposite side AB will be virtually indistinguishable from the arc length AC. That is,
An angle of 1 radian Note that an angle of 1 radian is a central angle whose subtending arc is equal in length to the radius. 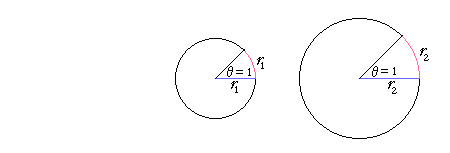
That is often cited as the definition of radian measure. Yet it remains to be proved that an arc equal to the radius in one circle, will subtend the same central angle as an arc equal to the radius in another circle. The main theorem cannot be avoided. (Moreover, although we can define an "angle of 1 radian," does such an angle exist? Can we know it? Is it possible to construct it?) Problem 1.
a) radius? Take π
arc is approximately three fifths of the radius. b) If the radius is 15 cm, approximately how long is the arc?
Problem 2. In a circle whose radius is 4 cm, find the arc length intercepted by each of these angles. Again, take π
d) 2π. (Here, the arc length is the entire circumference!)
Problem 3. In which quadrant of the circle does each angle, measured in radians, fall?
figure above.) Therefore, θ = 2 falls in the second quadrant.
figure above.) Therefore, θ = 5 falls in the fourth quadrant.
less than 2¼: 6.28 + 6.28 = 12.56. (See the figure above.) Therefore, θ = 14 falls in the first quadrant. Proof of the theorem In any circles, the same ratio of arc length to radius 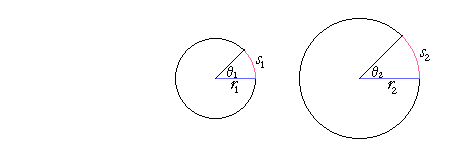
If, proportionally,
then θ1 = θ2. For,
implies, on dividing each side by 2π, 
But 2πr is the circumference of each circle. And each circumference is an "arc" that subtends four right angles at the center. Arcs, moreover, have the same ratio to one another as the central angles they subtend. (Theorem 16.) Therefore, 
and 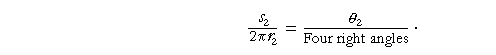
Hence, according to line (1), 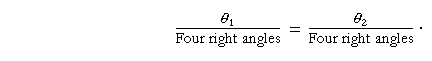
This implies θ1 = θ2. Therefore, the same ratio of arc length to radius determines a unique central angle that the arcs subtend. Which is what we wanted to prove. Next Topic: Analytic Trigonometry and the Unit Circle www.proyectosalonhogar.com |
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
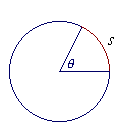
 That ratio of the circumference of a circle C to the radius r -- 2
That ratio of the circumference of a circle C to the radius r -- 2