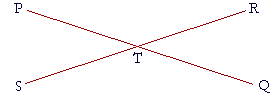
Book I. Proposition 15Problems Back to Proposition 15. 1. PQ, RS are straight lines that intersect at T. Name two pairs of
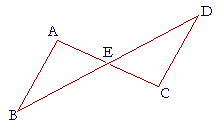
To see the answer, pass your mouse over the colored area. Angles PTR, STQ, and angles RTQ, PTS. 2. a) State the hypothesis of Proposition 15. Two straight lines intersect one another. 2. b) State the conclusion. The vertical angles are equal. 2. c) Practice Proposition 15. 3. Straight lines AC, DB intersect at E, at which both lines are bisected.
Prove that AB is equal to DC, and that angle A is equal to angle C.
By hypothesis, AE is equal to EC, and DE is equal to EB; Table of Contents | Introduction | Home Please make a donation to keep TheMathPage online. Copyright © 2006-2007 Lawrence Spector Questions or comments? E-mail: themathpage@nyc.rr.com |